asinh() math function to get inverse hyperbolic sin of x input number in radian in Python

asinh(x) returns inverse hyperbolic sin of x , the input number x is in radian.

import math
print(math.asinh(1))       # 0.8813735870195429
print(math.asinh(1.5))     # 1.1947632172871094
print(math.asinh(5))       # 2.3124383412727525

Note that all the inputs are in radian.
With negative input numbers

import math
print(math.asinh(-1))    #  -0.8813735870195429
print(math.asinh(-8.1))  #  -2.788800040920179

Inputs in degree

We can convert radian value to degree and use the same

import math
in_degree = 60
in_redian = math.radians(in_degree)
print(math.asinh(in_redian)) # 0.9143566553928859

Output

0.9143566553928859

1 radian = 57.2957914331 degree
1 degree = 0.0174533 radian

Drawing graph of asinh()

We will use Matplotlib to generate graph of asinh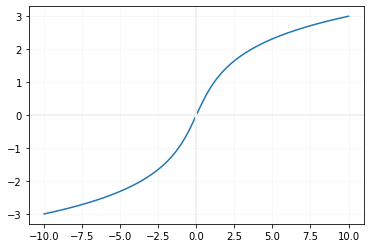 $asinh graph$

import matplotlib.pyplot as plt
x=[]
y=[]
i=-10
while (i<=10):
    x.append(i)
    y.append(math.asinh(i))
    i=i+0.1
plt.plot(x,y)
plt.axvline(x=0.00,linewidth=2, color='#f1f1f1')
plt.axhline(y=0.00,linewidth=2, color='#f1f1f1')
plt.grid(linestyle='-',
    linewidth=0.5,color='#f1f1f1')
plt.show()

Math Functions copysign() Python- Tutorials

Subscribe to our YouTube Channel here